See Thirds
Keely
The Neutralization of Magnets
"Thus, either present elements are the true elements, or else there is the probability before us of obtaining some more high and general power of nature, even than electricity, and which at the same time might reveal to us an entirely new grade of matter, now hidden from our view and almost from our suspicion. - [FARADAY]
Question. How can a magnet be robbed almost instantaneously of its magnetic power?
Answer. The peculiarity of the sympathetic conditions which conserve a magnet to polar and anti-polar currents of the earth, prove perfect sympathetic equation between reception and distribution in that part of the electrical field which is classified, in my system, as interatomic vibratory oscillation.
This oscillation represents, in its corpuscular field of action, an alternating wave-motion of one hundred and twenty-eight thousand four hundred vibratory exchanges per second, between polar reception and depolar distribution, thus establishing its perfect sympathetic concordance to that third of the electric triple stream which represents the sixths in vibratory sympathetic physics. The sympathetic action of the magnet, when electrically sensitized, becomes subservient to polar attraction as a medium through which a portion of its flow is diverted; no longer latent, but highly active as long as its magnetic sympathy (as electrically induced) continues, and it will then associate itself with every medium in nature in which this element exists in its latent state, from steel to oxygen at a low temperature.
We have now reached a starting-point from which to obtain a conception of the manner in which a magnet can be neutralized, that is, robbed of its coincident unity, or subservience to polar negative attraction." [The Operation of the Vibratory Circuit]
"Keely has discovered and was the first to demonstrate that electricity has never been handled; that it is not merely a force or a form of energy, - that it is matter; and that what we call electricity, and have diverted for commercial use in electric lighting, is but one of the triune currents, harmonic, enharmonic, and diatonic, which are united in pure electricity; that the enharmonic current seems to be sympathetically and mysteriously associated with the dominant current; and that the dominant current can no more be brought under control than can the lightning itself. The diversion of the dominant current would mean destruction to any mechanical medium used for that purpose, and death to the operator. The intense heat evolved by the electric stream Keely attributes to the velocity of the triple subdivision at the point of dispersion, as each triple seeks its medium of affinity. Sudden unition induces the same effect; but demonstration shows that the concentration of this triple force is as free of percussion as is the breath of an infant against the atmosphere; for the three currents flow together as in one stream, in the mildest sympathetic way, while their discharge after concentration is, in comparison to their accumulation, as the tornado's force to the waft of the butterfly's wing. The enharmonic current of this triple stream, Keely thinks, carries with it the power of propulsion that induces disturbance of negative equilibrium; which disturbance is essential to the co-ordination of its flow, in completing the triune stream of electricity. When this fluid is discharged from the clouds, each triplet or third seeks its terrestrial concordant, there to remain until that supreme law which governs disturbance of equilibrium again induces sympathetic concordant concentration, continuing to pass through its evolutions, positively and negatively, until the solar forces are expended." [Keelys Contributions to Science]
"The gravital flow comes, in this system, under the order of the sympathetic concordant of the 9ths, and belongs to that third of the triune combinations called polar propulsive.
"Magnetism is polar attraction.
"Gravity is polar propulsion.
"Both magnetism and gravity can be accelerated by the proper medium of sympathetic vibratory influences." [Keely and His Discoveries, Chapter 19]
Keely does not give an analysis of the structure of the etheric, but from the fact that he was able to subdivide it through the same process of "triple subdivision" into "interetherons" we may assume that three interetherons, each with its etheric capsule whirling about it, existed within the envelope of the etheron, vibrating with an oscillatory frequency greater than any of the lower subdivisions.
The fundamental mode of vibration changes as we reach the fifth subdivision, to the dominant, the diatonic third of the mass chord, which controls the vibratory states of both etheron and interetheron. The awful might concealed in the depths of the etheric and interetheric subdivisions utterly transcends anything Science has ever known. Even the theoretical energy value of radium now accepted by Science, pales into insignificance in comparison to the energy value of an equal amount of water subdivided to the etheric or interetheric state. [Snell Manuscript - The Book]
Music - The interval between a fundamental tone and the third diatonic tone above.
The interval comprised by two notes written on adjacent lines or spaces. A major third has two whole tones, a minor third a tone and a semitone, and a diminished third a whole tone.
The mode of a triad is determined by its third, as is the mode of a scale, since the sixth and seventh degrees are treated as variable in the harmonic minor and melodic minor scales. The diminished third is most often used as the inversion of the AUGMENTED SIXTH, INTERVAL, IMPERFECT INTERVAL [Westrup, J.A., Harrison, F. L.; Collin's Music Encyclopedia; William Collins Sons, & Co., Ltd., London, 1959]
1) Major Third 5:4
2) Minor Third 6:5
2.1) Augmented Third 125:96
2.2) A major third consists of four semitones, a minor third of three. A major tone is the whole tone having the ratio 8:9; a minor tone, that having the ratio 9:10. Intervals have had the term major applied to them in a conflicting manner.[Stainer, John; Barrett, W.A.; A Dictionary of Musical Terms; Novello, Ewer and Co., London, pre-1900]
3) Both occur for the first time in the third octave.
4) Are probably resultant tone, summation tone or compound tone.
5) Any two thirds make a Fifth, of which there are several:
| Diminished Fifth | 64:45 | |||||
| Diminished Fifth | 36:25 | |||||
| Perfect Fifth | 3:2 | |||||
| Augmented Fifth | 25:16 | |||||
6) They play a key role in the simplest chords: Triads, of which there are three types
a) Major
b) Minor
c) Diminished
(The material of which music is made is tone, in recognizable, orderly chord groups. The simplest chord group is the Triad, or three tone chord. The Triad always consists of fundamental (root), third and fifth. A Triad may be constructed upon every degree of the scale, Major and Minor. Upon the Major Scale tones the Triads of the key, in C Major, are shown above. These seven Triads occur in exactly the same form in every Major Key. There are three different Triad groupings in the above:
Major Triad: Major 3rd and Perfect 5th on the 1st, 4th and 5th degrees.
Minor Triad: Minor 3rd and Perfect 5th on the 2nd, 3rd and 6th degrees.
Diminished Triad: Minor 3rd and Diminished 5th on the 7th degree.)
DEFINITIONS
1) The interval between a fundamental tone and the third diatonic tone above.
2) The interval comprised by two notes written on adjacent lines or spaces. A major third has two whole tones, a minor third a tone and a semitone, and a diminished third a whole tone. The mode of a triad is determined by its third, as is the mode of a scale, since the sixth and seventh degrees are treated as variable in the harmonic minor and melodic minor scales. The diminished third is most often used as the inversion of the AUGMENTED SIXTH, INTERVAL, IMPERFECT INTERVAL. Collin's Music Encyclopedia, 1959
3) Progressive Thirds: The ultimate test of a good temperament on any piano is a smooth change of the beat rates as progressive thirds are played - both in the bearings octave, and outside it. (no reference)
4) Diatonic Thirds: "The sympathetic acoustic impulses are: the DOMINANT - a diatonic third - the HARMONIC - the connective "sixth" - and the ENHARMONIC - or diminished seventh - which Keely calls a ninth - inducing "infinite trajective velocity" or "neutral radiation" from neutral centers." The Snell Manuscript
Third, Neutral
neutral 3rd, neutral third, n3
An interval intermediate in size between the Major Third and the Minor Third.
Neutral Thirds measure about 350 cents (¢) and typical examples are 11/9 (347¢), 27/22 (355¢) and 16/13 (359¢). (from John Chalmers, Divisions of the Tetrachord)
Thirds, Sixths and Ninths
Fri, 15 Dec 2000
On the one hand Keely talks about musical intervals - on the other hand he mixes in references to the orders of matter and energy, volume and divisions of chords. Which is which and when? To me, the above does NOT refer solely to musical chords. I've said this before. But instead represents a wider view of order, dynamics and structure. For instance if we view the nine strings of the CEG chord as three sets of three vibrating on 1, 2 and 3 octaves we get the possibility of the first third of the WHOLE NINE STRING CHORD being octave one, the second third being octave two and the third third as octave three of this nine string chord. The nine string chord is seen to be composed by thirds of the whole. These represent the molecular, atomic and etheric realms or levels of the matter and energy scheme being three major thirds of the whole of nature. Therefore the first third is the enharmonic (earthy earth; i.e.; terrestrial) the second third represents the harmonic sixths (Russell's fulcrum?), and the third third represents the infinite ninths (celestial). Rotation is the result of a conflict between the first third (terrestrial) and the third third or ninths (celestial) as given above. I've included below quotes from Keely on his rotating sphere. If you've read Russell you will see DIRECT correlations between the two men expressing the SAME concept of rotation occurring in this manner - and ONLY in this manner. If you have not read Russell you may not see this. This being the case the CEG chord is the centralizing chord to the center (fig. 1) while the BfDG chord is the dispersive chord (fig. 2) - each chord representing the in and out FLOWING STREAMS (fig. 5) to and from the center. Keep in mind the dynasphere represents a faithful micro/macrocosm of the universal forces as depicted on the "Universal Cosmology - Genesis" page I recently put together. The 24 resonators, tuned to musical thirds, placed in eight triplets around the Ring of Resonation are coincident to each of the three-sided corners (fig. 17) of the cube of celestial dispersion realm (fig. 2) while the second ring of resonation inside the sphere are tuned to Bf keynote of the earth or spherizing element in nature. So much more needs to be done yet with all this but we are hot on the trail. [Dale.]
Thirds, Controlling
"The first third is controlled by the molecular, the next progressive third by the atomic and the highest third by the etheric." [Keely and His Discoveries]
Thirds and Triads
A TRIAD is composed of two thirds. There are two kinds of thirds: a Minor (mn) and a Major (mj). These may be combined in two ways to create a Triad: (mn+mj) and (mj+mn). The former creates a Minor Triad (5mn) while the second creates a Major Triad (5mj). There are several issues being sorted out here. One is how to count steps and/or intervals. This is a real problem as far as I can see especially when trying to computerize all these factors. The first column under "THIRDS" is read thusly: "A?C" reads "A? to C", etc. The accented notes are not differentiated between A# and B? for instance; being the same note.
THIRDS
| Interval | Step | Mode | ||||
| A? to C | 2 | Major Third | ||||
| A to C | 1.5 | Minor Third | ||||
| A to C# | 1.5 | Minor Third | ||||
| A# to D? | 1.5 | Minor Third | ||||
| B? to D | 2 | Major Third | ||||
| B to D | 1.5 | Minor Third | ||||
| B to D# | 2 | Major Third | ||||
| C to B? | 2 | Major Third | ||||
| C to E | 2 | Major Third | ||||
| C to D# | 1.5 | Minor Third | ||||
| C# to E | 1.5 | Minor Third | ||||
| D? to F | 2 | Major Third | ||||
| D to F | 1.5 | Minor Third | ||||
| D to F# | 2 | Major Third | ||||
| D# to F# | 1.5 | Minor Third | ||||
| D# to G | 2 | Major Third | ||||
| E to G | 1.5 | Minor Third | ||||
| E to G# | 2 | Major Third | ||||
| F to A | 2 | Major Third | ||||
| F to A? | 1.5 | Minor Third | ||||
| F# to A | 1.5 | Minor Third | ||||
| F# to A# | 2 | Major Third | ||||
| F# to B? | 2 | Major Third | ||||
| G to B | 2 | Major Third | ||||
| B to B? | 1.5 | Minor Third | ||||
| G# to B | 1.5 | Minor Third | ||||
TRIADS
| C E G | = | 5mj | = | 3mj+3mn | = | CE+EG |
| C E? G | = | 5mn | = | 3mn+3mj | = | CD#+D#G |
| D F A | = | 5mn | = | 3mn+3mj | ||
| D F# A | = | 5mj | = | 3mj+3mn | ||
| E G B | = | 5mn | = | 3mn+3mj | ||
| E G# B | = | 5mj | = | 3mj+3mn | ||
| F A C | = | 5mj | = | 3mj+3mn | ||
| F A? C | = | 5mn | = | 3mn+3mj | ||
| G B D | = | 5mj | = | 3mj+3mn | ||
| G B? D | = | 5mn | = | 3mn+3mj | ||
| A C E | = | 5mn | = | 3mn+3mj | ||
| A C# E | = | 5mj | = | 3mn+3mn | ||
The last triad is a Diminished triad and before the third is raised it is important to raise the fifth:
| B D F | = | dim | = | 3mn+3mn | ||
| B D F# | = | min | = | 3mn+3mj | ||
| B D# F# | = | maj | = | 3mj+3mn | ||
Ramsay
in their birthplace - F A C, C E G, G B D. Indeed in their birth not only is it so, but still further, the top note of the first chord is the root and generator of the third. They are linked in generative continuity. [Scientific Basis and Build of Music, page 49]
a minor third. So by adding the middle of the minor dominant, G, but made G#, that the third so produced may be a minor third, according to the nature of the chromatic chords, we have on this minor side of the chord G#, B, D, F, which we may call its minor form, inasmuch as the semitone of its second minor third is the one, B-C, which genetically arises in the minor genesis; and inasmuch as it has also received its supplemental G# from the minor dominant. How shall we find its complement on the other side? We have seen that D, the Janus-faced center of this triad, B, D, F, looks, as D27, toward the major also; it has already F in common with the major subdominant. The very next step is to the middle of this chord, A. Middles, we have just seen, are ever ready to accommodate themselves; and this minor third triad claims that A be flattened, for on this side also, though its major side, it must have a minor third; so by adding the middle of the major subdominant, A, but made A?, according to the nature of chromatic intervals, that this F-A? also may be a minor third; and now we have it as B, D, F, A?, which we may call its major form, inasmuch as the semitone of its minor third, E-F, is the one which genetically arises in the major genesis, and inasmuch as it has now received its supplemental A? from the major subdominant. This, then, is the chromatic chord in its native place, and in its native constitution; a 4-note chord, wholly of the minor thirds. It will be observed that it has now, in its two forms, divided the octave into minor thirds - 4 minor thirds, so it is very much at home anywhere in the octave; indeed it is at home everywhere - G#, B, D, F, A?. And as every diatonic common chord in music is constituted of materials found in the octave of notes, it cannot be far from a chromatic chord in some one of its forms. [Scientific Basis and Build of Music, page 55]
This great genetic scale, the all-producer, the all-container, extends over six octaves on each side; for it is not till high in the sixth octave we get B in the major, and it is not till low in the sixth octave that we get F in the minor. It is in the fifth octave, however, that the note which is the distinctive mark of the masculine and feminine modes is generated. D27 in the major, and D26 2/3 in the minor, distinguishes the sex of the modes, and shows which is the head and which the helpmeet in this happy family.2 On the major side F, the root of the subdominant chord, that is the chord which is a fifth below the key-note C, is the root of all. This is the beginning of this creation. If we call the vibration-number of F one, for simplicity's sake, then F1 is multiplied by 3 and by 5, which natural process begets its fifth, C, and its third, A; this is the root, top, and middle of the first chord. From this top, C3, grows the next chord by the same natural process, multiplying by 3 and by 5; thus are produced the fifth and third of the second chord, G and E. From the top of this second chord grows the third and last chord, by the repetition of the same natural process; multiplying G9 by 3 and by 5 we [Scientific Basis and Build of Music, page 66]
The peculiar effects are exhibited when the chord-scale is next set forth. We have seen that there are six chords evolved in the Genesis upward and downward, 3 in the major form and 3 in the minor. In the fifths of the minor the semitone is always in the lower third, occurring between the second and third in the subdominant and tonic, and between the first and second in the dominant chord; whereas in the major it is always in the upper third, between the fourth and fifth in the subdominant, and between the third and fourth in the tonic and dominant chords. While the thirds which the fifths contain are thus so varied, the fifths themselves have always one magnitude, whether major or minor. [Scientific Basis and Build of Music, page 68]
The varied effect of position in chords. When a chord stands as C E G C, having its root also at the top, it has its softest, dullest, most united effect; it is undramatic, with little contrast. When it stands as E G C E, having its third at the top and bottom, it has a more ticklish, interesting, far-away effect. In reveries composers often finish thus, as if it had vanished - an unsettled effect. When it stands as G C E G, with its top at top and bottom, it has its most dominant character - loud, swelling. In the position C E G C it stands mixingly with the subdominant C E f G a C, and in this its first position its unseen filling in is chiefly from the region of gravity; hence its soft, grave, dull, heavy effect; and it passes very easily to the subdominant chord. When it stands as G C E G it stands mixingly with the dominant G b C d E G, and has its third position and most brilliant effect and uprising, for its unseen filling in is then chiefly from the region of levity; and it passes easily to the dominant chord. When in its second position, its middle position E G C E, its unseen filling in is mixingly both subdominant and dominant, E f G a b C d E; it has then its most interesting and puzzling effect; on the one hand its softest, dullest, and one-est, on the other hand its most brilliant effect, as if it would at once both sink and soar. [Scientific Basis and Build of Music, page 72]
N.B. - The sharp comes here by the prime 5, and the comma by the prime 3. Now we have the key of G provided for;-
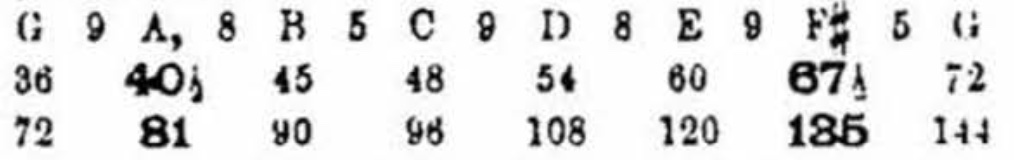
These are two octaves of the scale of G. G A B, which in the scale of C was an 8-9-comma third, must now take the place of C D E, which in C was a 9-6-comma [Scientific Basis and Build of Music, page 82]
third; and in order to do so, A has been mathematically raised a comma, which makes G A B now a 9-8 comma third. E F G, which in the scale of C was a 5-9 comma third, must now take the place of A B C in the scale of C, which was a 9-5 comma third; and in order to do so, F is mathematically raised 4 commas, and must be marked F#; and now the interval is right for the scale of G. E F# G is a 9-5 comma interval. This mathematical process, in the majors, puts every scale in its original form as to vibration-numbers; but since the same letters are kept for the naming of the notes, they must be marked with commas or sharps, as the case may be. In the Sol Fa notation such marks would not be necessary, as Do is always the key-note, Ray always the second, and Te always the seventh. [Scientific Basis and Build of Music, page 83]
THIS plate is a Pendulum illustration of the System of musical vibrations. The circular lines represent Octaves in music. The thick are the octave lines of the fundamental note; and the thin lines between them are lines of the other six notes of the octave. The notes are all on lines only, not lines and spaces. The black dots arranged in these lines are not notes, but pendulum oscillations, which have the same ratios in their slow way as the vibrations of sounding instruments in the much quicker region where they exist. The center circle is the Root of the System; it represents F1, the root of the subdominant chord; the second thick line is F2, its octave; and all the thick lines are the rising octaves of F, namely 4, 8, 16, 32, and 64. In the second octave on the fifth line are dots for the three oscillations which represent the note C3, the Fifth to F2, standing in the ratio of 3 to 2; and the corresponding lines in the four succeeding Octaves are the Octaves of C3, namely 6, 12, 24, and 48. On the third line in the third Octave are 5 dots, which are the 5 oscillations of a pendulum tuned to swing 5 to 4 of the F close below; and it represents A5, which is the Third of F4 among musical vibrations. On the first line in the fourth Octave are 9 dots. These again represent G9, which stands related to C3 as C3 stands to F1. On the seventh line of the same octave are 15 dots; these represent the vibrations of E15, which stands related to C3 as A5 stands to F1. On the sixth line of the fifth Octave are 27 dots, representing D27, which stands related to G9 as G9 stands to C3, and C3 also to F1; it is the Fifth to G. And last of all, on the fourth line of the sixth Octave are 45 dots, representing B45, which, lastly, stands related to G9 as E15 stands to C3, and A5 to F1; it is the Third to this third chord - G, B, D. The notes which arise in each octave coming outward from the center are repeated in a double number of dots in the following Octaves; A5 appears as 10, 20, and 40; G9 appears as 18 and 36; E15 appears as 30 and 60; D27 appears as 54; and last of all B45 only appears this once. This we have represented by pendulum oscillations, which we can follow with the eye, the three chords of the musical system, F, A, C; C, E, G; and G, B, D. C3 is from F1 multiplied by 3; G9 is from C3 multiplied by 3; these are the three Roots of the three Chords. Their Middles, that is their Thirds, are similarly developed; A is from F1 multiplied by 5; E15 is from C3 multiplied by 5; B45 is from G9 multiplied by 5. The primes 3 and 5 beget all the new notes, the Fifths and the Thirds; and the prime 2 repeats them all in Octaves to any extent. [Scientific Basis and Build of Music, page 102]
mathematical genesis, as seen in its D being a comma higher than that of the minor. This gravity and buoyancy of the modes is a striking feature of them. In the Thirds it is different from the Fifths; the larger hemisphere of each third seems gravitating toward the center of the tonic chord. The area of the scale has then the aspect of a planet with its north and south poles, and pervaded by a tendency towards the center; the center itself being neutral as to motion. [Scientific Basis and Build of Music, page 107]
When Leonhard Euler, the distinguished mathematician of the eighteenth century, wrote his essay on a New Theory of Music, Fuss remarks - "It has no great success, as it contained too much geometry for musicians, and too much music for geometers." There was a reason which Fuss was not seemingly able to observe, namely, that while it had hold of some very precious musical truth it also put forth some error, and error is always a hindrance to true progress. Euler did good service, however. In his letters to a German Princess on his theory of music he showed the true use of the mathematical primes 2, 3, and 5, but debarred the use of 7, saying, "Were we to introduce the number 7, the tones of an octave would be increased." It was wise in the great mathematician to hold his hand from adding other notes. It is always dangerous to offer strange fire on the altar. He very clearly set forth that while 2 has an unlimited use in producing Octaves, 3 must be limited to its use 3 times in producing Fifths. This was right, for in producing a fourth Fifth it is not a Fifth for the scale. But Euler erred in attempting to generate the semitonic scale of 12 notes by the use of the power of 5 a second time on the original materials. It produces F# right enough; for D27 by 5 gives 135, which is the number for F#. D27 is the note by which F# is produced, because D is right for this process in its unaltered condition. But when Euler proceeds further to use the prime 5 on the middles, A, E, and B, and F#, in their original and unaltered state, he quite errs, and produces all the sharpened notes too low. C# for the key of D is not got by applying 5 to A40, as it is in its birthplace; A40 has already been altered for the key of G by a comma, and is A40 1/2 before it is used for producing its third; it is A40 1/2 that, multiplied by 5, gives C#202 1/2, not C200, as Euler makes C#. Things are in the same condition with E before G# is wanted for the key of A. G# is found by 5 applied to E; not E in its original and unaltered state, E30; but as already raised a comma for the key of D, E30 3/8; so G# is not 300, as Euler has it, but 303 3/4. Euler next, by the same erroneous methods, proceeds to generate D# from B45, its birthplace number; but before D# is wanted for the key of E, B has been raised a comma, and is no longer B45, but B45 9/16, and this multiplied by 5 gives D#227 13/16, not D225, as Euler gives it. The last semitone which he generates to complete his 12 semitones is B?; that is A#, properly speaking, for this series, and he generates it from F#135; but this already altered note, before A# is wanted for the key of B, has been again raised a comma [Scientific Basis and Build of Music, page 107]
Hughes
ON a keyed instrument only twelve are major key-notes, but as the double tones C#-D? and F#-G? are roots, there are fourteen different chords. The fourteen that are roots are written in musical clef. As an example of the major chords in the different keys, we may examine those in the key of C. A major fifth includes five out of the seven of its key; with the third or central note it is the threefold chord, or fourfold when the octave note is added. Including the silent key-notes, a threefold chord embraces eight, or, counting the double tones, not including E#, eleven. The first and second chords of the seven of the harmony are perfect major chords in the key of C; the central note of the third chord, being #C-?D, is a discord. The first pair of fifths in the scale, with its central note, is a chord of the key; if we include the octave, the last pair of fifths, with its central note, is the same chord an octave higher than the lowest chord of the seven. Of the chords written in musical clef of the twelve keys, the octave chord is only written to C, the seven of each having two chords and the scale one, thirty-six in all, or forty-eight if the octave chords are added. Notice how the chords of each seven and the chord of its scale are altered. [Harmonies of Tones and Colours, Diagram V - The Chords of the Twelve Major Keys, page 27a]
See Also
12.07 - Keelys Thirds Sixths and Ninths
14.04 - Thirds as Currents
14.05 - Thirds as Differentiations
14.07 - Thirds in Magnetic Action
14.08 - Thirds as Assimilatives
14.10 - Thirds as Ratios within a Whole
14.28 - Thirds as Polar and Depolar Parameters
16.08 - Polar Link in Thirds
7.12 - Third
Chord
Figure 11.01 - Octave composed of Equal Thirds and Triads
harmonical triad
Interval
Minor Triad
note in common
Part 14 - Keelys Mysterious Thirds Sixths and Ninths
perfect triad
Rhythmic Balanced Interchange
Table 1 - Relations of Thirds
Table 14.01 - All phrases in HyperVibes containing the term thirds
Table 14.02 - Neutral Thirds - Energy Radiates from Center - Force Contracts to Center
one third
third
thirds
two-thirds of one-third
two-thirds of the one-third
Three
Trimer
Triplet
Universal Heart Beat
