Thickness - (Volume, Sphere)
In graphic Figure 12.09 - Dimensions and Relationships it is clear:
Relative Volume
4+ = 1/8 of 3+ or 3+ = 8 X 4+ or 81
3+ = 1/8 of 2+ or 2+ = 8 X 3+ or 82
2+ = 1/8 of 1+ or 1+ = 8 X 2+ or 83
Numeric Progressions (units)
1st Dimension = Linear = 1, 2, 4, 8.. (Doubling, nX2)
2nd Dimension = Area = 1, 4, 8, 64.. (Squaring, n2)
3rd Dimension = Volume = 1, 8, 64, 512.. (Cubing, n3)
Volumes
Cube Volume = 1 = 13 = 1 on a side
Cube Volume = 2 = cube root of 2 = 1.259922 on side
Cube Volume = 4 = cube root of 4 = 1.587403 on side
Cube Volume = 8 = cube root of 8 = 2 on sidetherefore
Wavelengths and Frequencies - Octave Relations of Russell's Indig Number System
| Indig | Vol. Units | Vol. Calc | Wavelength | Example | Octave | Note |
4 | 1 | 13 | 1 | 1 cps | 4 | G as 4th octave |
3 | 8 | 23 | 2 | 1/2 cps | 3 | F as 3rd octave |
2 | 64 | 43 | 4 | 1/4 cps | 2 | E as 2nd octave |
1 | 512 | 83 | 8 | 1/8 cps | 1 | D as 1st octave |
0 | C## non-octave |
Table 12.02.01 - Wavelengths and Frequencies
Showing linear versus geometric progressions as also other types of progressions (counting methods or scales).
See Also
arithmetical progression
Frequency
Geometrical Progression
Laws of Being
progression
Ratio
Reciprocal
Reciprocating Proportionality
Square Law
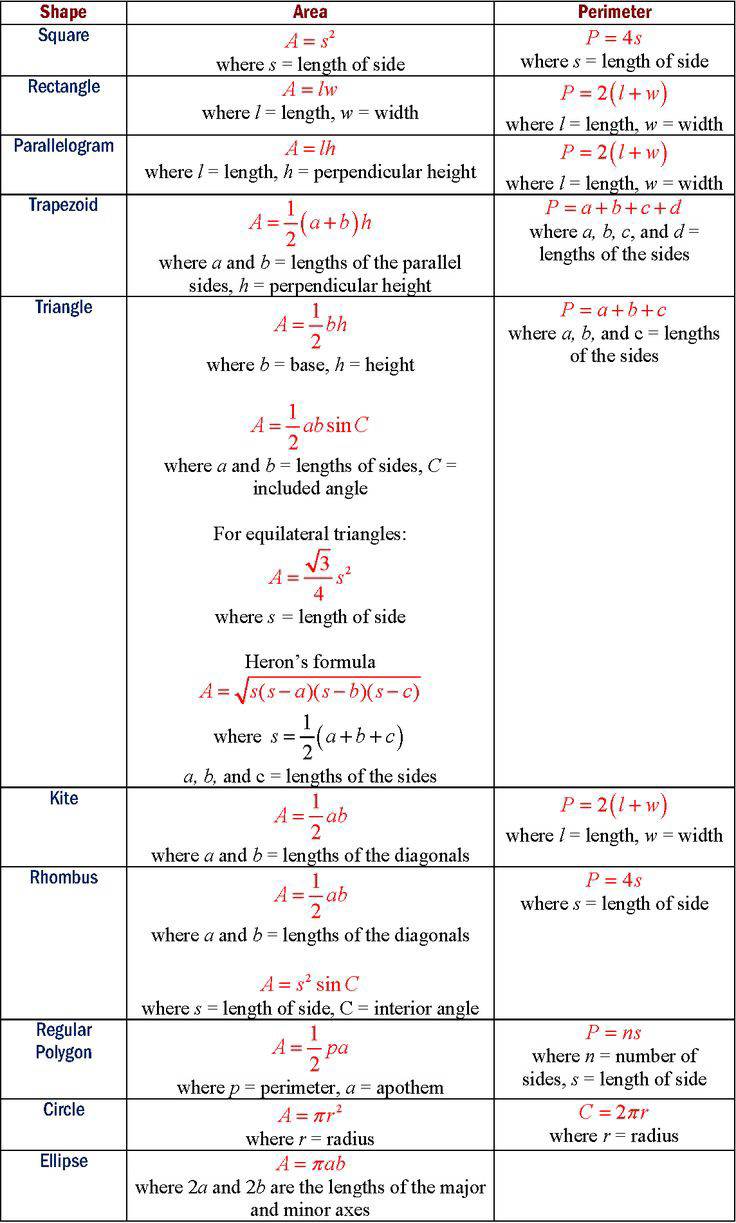
Table 12.02 - Length Area and Volume Math
Tone
Volume
wave number
Wavelength
12.00 - Reciprocating Proportionality
12.18 - Multiple Octave Progression
References
Calculate various Properties of a Cylinder
See Also
Area
Cube
Length
Sphere
Volume
