Negative Covariance [See Conjugate Variables, reciprocal] is seen in many of the Russell graphics and is found throughout SVP. This principle is easily seen, as an example, in the dynamic relationship between the Square Law and Inverse Square Law. Russell and I have used the term 'reciprocal' to denote 'covariance' or covariant conditions - also referred to as Conjugate Variables in thermodynamics.
The simplest way to see this equal and opposite exchange of forces is through a child's seesaw.

Covariant Seesaw Motions (click to enlarge) |

Covariant Seesaw Motions (click to enlarge) |
Russell presented this same idea in many of his illustrations especially this one:
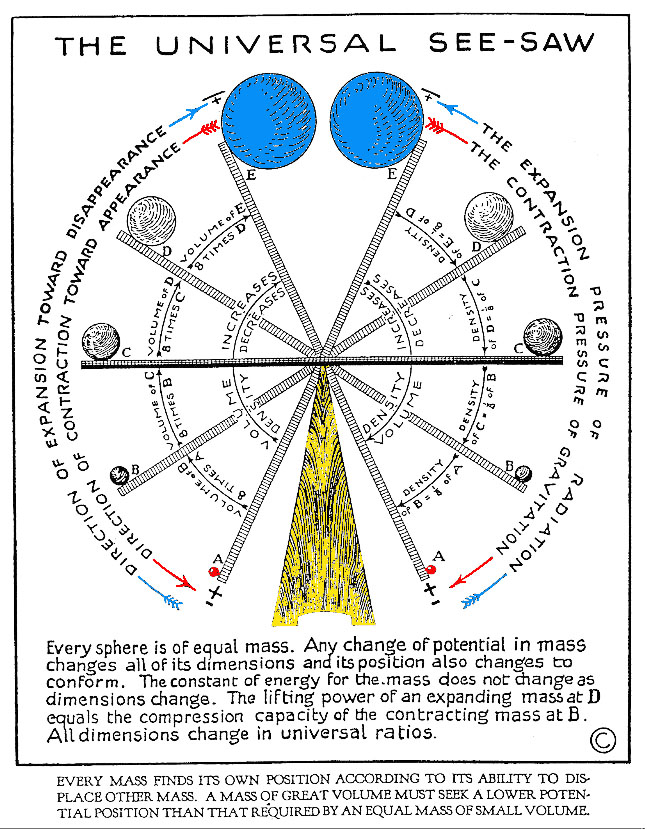
Universal Seesaw (courtesy University of Science and Philosophy) (click to enlarge)
Examples:
"The Law of Love is rhythmic balanced interchange between all things. Upon the law of balanced interchange, this entire reciprocal universe is motivated with such exactness of balance that astronomers can calculate the positions of planets and suns to the split second. In this wise, the universe is dependable. It observes the Law. It cannot do otherwise where God controls all things." [Russell, The Universal One, Preface]
"Every dimension has its opposites, and every change in preponderance from one opposite to the other simultaneously changes every other one of the eighteen series of opposites to balance that change." [Russell, The Universal One, Book 02 - Chapter 11 - Attraction and Repulsion]
"All dimensions simultaneously expand and contract in opposite directions of the same ratio. [Russell, The Universal One, Book 02 - Chapter 14 - Universal Mathematics - Universal Ratios]
Additional images and links: 3.13 - Reciprocals and Proportions of Motions and Substance 7.3 - Law of Love - Reciprocal Interchange of State on Multiple Subdivisions Figure 12.13 - Some Multi-Dimensional as Inverse and Direct Reciprocal Relationships Figure 13.14 - Equilibrium as Reciprocal Forces Rotation and Revolution are Reciprocals
