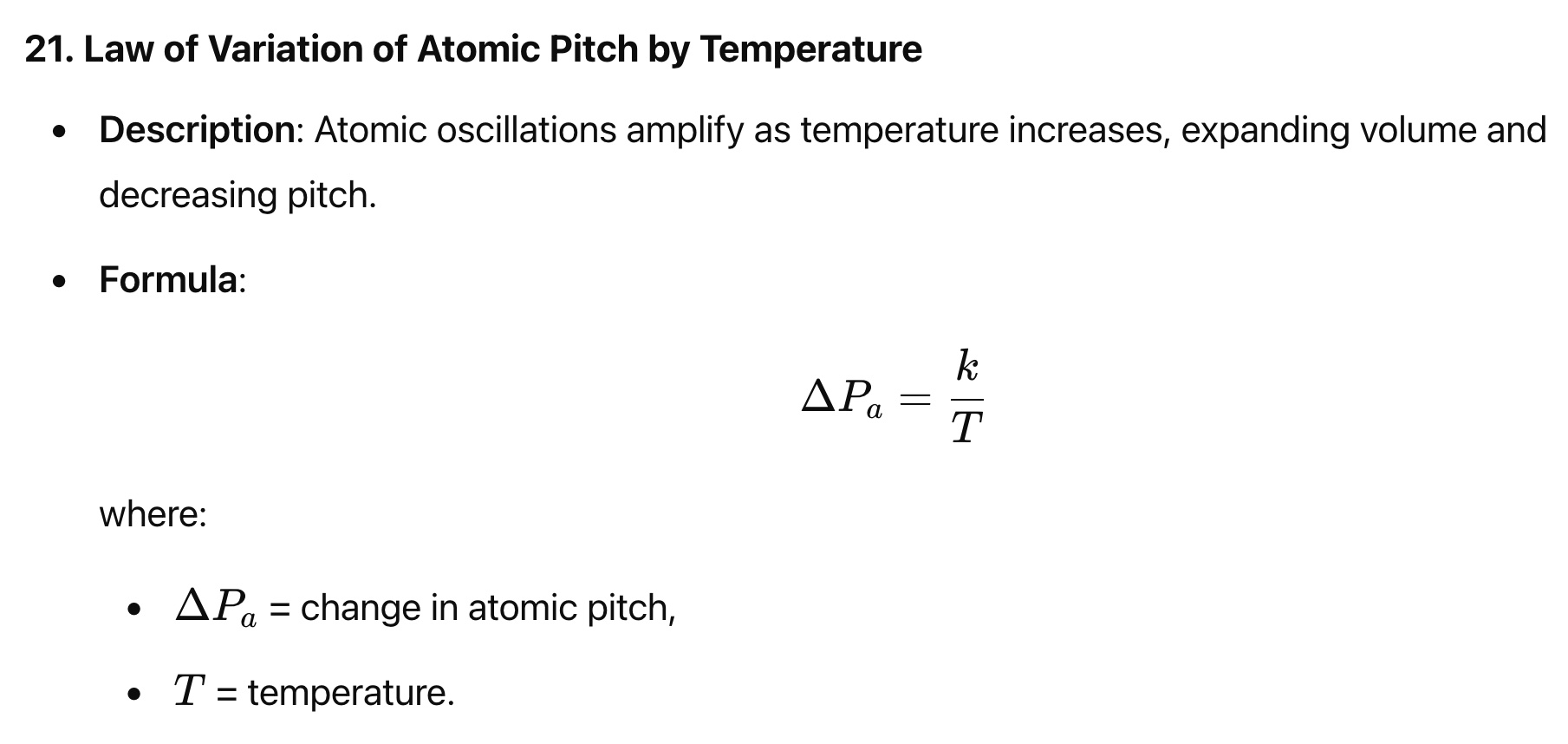
Law of Variation of Atomic Pitch by Temperature
"Atoms in chemical combination oscillate with increasing amplitude directly as the temperature, and simultaneously absorb overtones of higher harmonics, producing expansion of volume and diminution of pitch.
Rule: The gradual approach of the temperature of harmonic combination can be observed by mutually comparing superimposed spectra; chemical combination commences when the fundamental lines of each spectrum bear harmonic ratios by linear measurement." [Keely, 1893]
Commentary November, 2017
This is a fairly obvious law. Combining atoms into molecules increase in oscillation directly as temperature increase. The combination absorbs higher harmonics of overtones[1] (developed from intensification) creating a swelling or increase in volume due the expansion. As volume increases pitch decreases.
Frequencies, as measured by light spectra, can be determined by fundamental lines of the spectra. The elements combine in harmonic ratios[2] which can be determined by measurement.
"The Fraunhofer lines represent the silences, or the places of |invisible pitches between the luminous pitches of rad-energy. They cannot therefore be conveniently used as data from which to measure the fundamental pitches of the atoms undergoing examination. When a series of sound-pencils are projected upon a screen, they undergo a combination of overtones and undertones at the point of contact producing tones of a pitch either too low to be recognized by the human ear or too high to be called sound. The Fraunhofer lines are not therefore simply silences, but may be the higher invisible ultra-actinic rays. The fact is that some of the Fraunhofer lines are capable of producing a variety of chemical actions, when reflected and focalized. Observation thus far shows that these lines do not bear any definite ascertainable relation to the pitches producing them, but that they do bear some uniform relation from which the fundamental pitch could be determined cannot be doubted. The relation of the Fraunhofer lines to the luminous spectra are undoubtedly such as would enable one to compute the creative pitches producing them; but as yet no such determinations have been made. The accurate method of determining them is from the mutual relation of the harmonic pitches of the luminous spectra.
"A table representing the harmonic overtones and undertones of simple vibrations, and the resultant harmonics of associate vibrations, will be of great convenience in making these determinations.
"The natural unity of sonity lies above 1 per second, and below 2 per second, and for this reason the numbering of the octaves is accomplished by calling the end of the first octave No. 1 instead of No. 2.
"At the end of the twenty-first octave sono-thermity commences, and the bodies oscillating at this pitch are either correspondingly smaller by 1/8 than the preceding sonitic aggregates; or larger aggregates undergo vibration in submultiple portions of themselves. In either case the originating oscillation of sono-thermic pitch is that of an isolated or localized aggregation. This first class of forces, or first double gamut, is included within the range of about forty-three octaves. The bodies of the translatory pendulous motion and produce waves of the transverse form, while the bodies of the second gamut undergo internal nodal vibration and produce waves of a longitudinal form.
"Beyond the upper limit of the forty-third octave we reach bodies of a size (determined by the same method as in sonity) which we know to be about the size of an atom as approximately determined by various physicists to lie between eleven and twelve micromillimeters (hydrogen molecules), which gives the highest pitch of the known atoms, and from which can be roughly estimated the pitch of the heavier atoms. Starting with the approximate pitch of hydrogen as determined from its associate spectrum with oxygen, and working back to the size of the largest atoms, we again reach a pitch corresponding to the highest sono-thermic vibrations. Starting with the known temperature and pitch of a heated body, emitting definite rays of light, and working back to absolute zero, we again reach the pitch of the sono-thermic limit." Dashed Against the Rock, pg 77-78.
[1] See Law of Cycles
[2] See Bjerknes Effect, Law of Attraction
Return to Keelys Forty Laws
See Also
