Mass ratios for elementary particles
These numbers derive from the eigenvalues of the exceptional Jordan algebra. This is the algebra of 3x3 Hermitean matrices with octonions as entries. The chosen values of the octonions correspond to different elementary particles; these choices in turn are related to the left minimal ideals [spinors] of a Clifford algebra which represent the particles. The eigenvalues of these matrices are expected to inform us about mass ratios, and indeed they do.
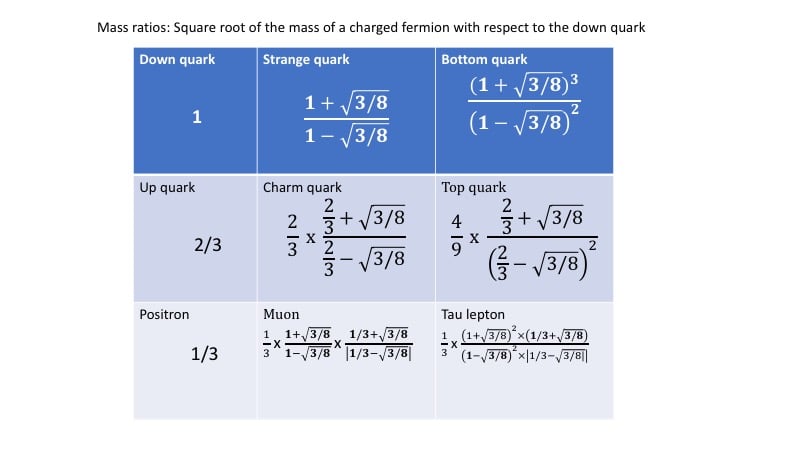
If you take the mass of a charged fermion, divide it by the mass of the down quark, and take the square root of the result, that number will be reproduced by the corresponding number shown in the table below. Within the known experimental uncertainty of the masses.
These mass ratios have the same fundamental status as quantized electric charge values 1/3, 2/3, 1. They constitute [perhaps the first ever] demonstration of mass quantization from first principles. These eigenvalues are the same as the ones which led to the derivation of the low-energy fine structure constant 1/137.xxx
So now we could say we know why the muon is 200 times heavier than the electron. It is because elementary particles are described by the octonions! [anon on FaceBook]